Introduction
Have you ever wondered how to determine the 300th digit in a repeating decimal sequence? The decimal 0.0588235294117647 is a recurring decimal, making it essential to understand how to identify a specific digit within its pattern. In this guide, we will explore decimal expansions, repeating sequences, and the precise method to find the 300th digit of 0.0588235294117647.
Understanding Decimal Expansions
Decimal numbers can be classified into terminating decimals and repeating decimals. A terminating decimal ends after a finite number of digits (e.g., 0.5 or 0.75), while a repeating decimal continues infinitely with a recurring sequence. The decimal 0.0588235294117647 belongs to the latter category, as it originates from a fraction that has a periodic pattern.
The Fraction Behind 0.0588235294117647
The decimal 0.0588235294117647 represents the fraction 1/17. When 1 is divided by 17, the result is a repeating sequence of 16 digits:
0.0588235294117647 0588235294117647 0588235294117647 ...
Understanding this pattern allows us to determine any specific digit in its decimal expansion.
Finding the 300th Digit of 0.0588235294117647
To find the 300th digit in this repeating sequence, follow these steps:
Step 1: Determine the Length of the Repeating Cycle
The decimal expansion of 1/17 has a repeating cycle of 16 digits.
Step 2: Use Modular Arithmetic
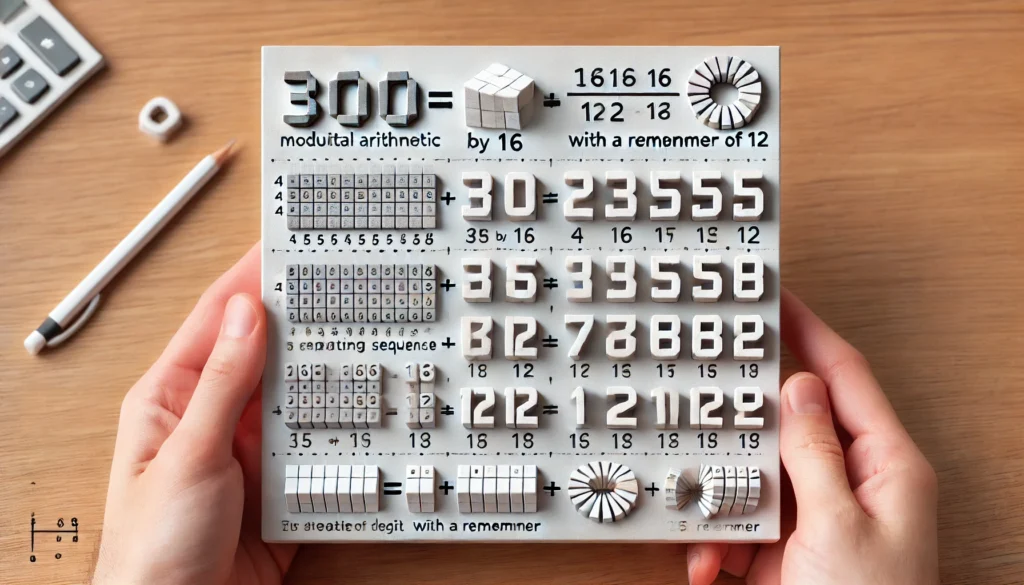
To locate the 300th digit, divide 300 by 16:
300 ÷ 16 = 18 remainder 12
Since the remainder is 12, the 300th digit corresponds to the 12th digit in the repeating sequence.
Step 3: Identify the 12th Digit
Looking at the repeating sequence:
0.0588235294117647
The 12th digit is ‘4’.
Thus, the 300th digit of 0.0588235294117647 is 4.
Real-World Applications of Repeating Decimals
Repeating decimals are more than just a mathematical curiosity; they have practical applications in various fields, including:
- Engineering & Physics – Used in precision measurements.
- Computer Science – Important in algorithms and floating-point arithmetic.
- Finance & Economics – Helps in calculating interest rates and periodic payments.
- Cryptography – Plays a role in encryption techniques.
- Statistics – Essential for probability calculations.
Common Misconceptions About Repeating Decimals
- Repeating Decimals Are Only in Fractions – While fractions often generate repeating decimals, irrational numbers like π also have infinite decimals, but without a repeating pattern.
- All Fractions Have a Repeating Pattern – Some fractions, like 1/2 or 3/4, terminate instead of repeating.
- A Longer Decimal Means a More Complex Fraction – The length of the repeating sequence depends on the denominator, not the fraction’s complexity.
Table: Key Facts About 1/17
| Property | Value |
|---|---|
| Fraction Form | 1/17 |
| Decimal Expansion | 0.0588235294117647 (repeats every 16 digits) |
| Repeating Sequence Length | 16 |
| 300th Digit | 4 |
Frequently Asked Questions (FAQs)
1. Can the 300th digit of other repeating decimals be found the same way?
Yes! The same modular arithmetic method applies to any repeating decimal.
2. How do I find the repeating cycle of any fraction?
Divide the numerator by the denominator and observe the repeating pattern in the decimal expansion.
3. What if the remainder is 0 when dividing by the cycle length?
If the remainder is 0, the digit is the last one in the repeating sequence.
4. Can a repeating decimal ever become a terminating decimal?
No, a repeating decimal remains infinite unless rounded for practical purposes.
5. Are there other fractions with a 16-digit repeating cycle?
Yes, fractions like 2/17, 3/17, etc., also have a 16-digit repeating sequence but with different starting points.
Conclusion
In this guide, we explored how to determine the 300th digit of 0.0588235294117647 using modular arithmetic and the properties of repeating decimals. By understanding the cycle length and applying simple division, we identified that the 300th digit is 4. Repeating decimals play a crucial role in various scientific and mathematical applications, making them an essential concept to grasp. Whether in engineering, finance, or computing, knowing how to handle repeating decimals enhances numerical precision and problem-solving skills.
RECOMMENDED ARTICLES
Dinsdale Shop Chair Cushions for Sale – The Ultimate Buying Guide
The Ultimate Guide to Table Cloth Pra Mosca
Puneeth Kamath Pegaworld: A Comprehensive Guide to Innovation and Digital Transformation
Ultimate Travel Guide: 68 Tetz Rd Chester NY to 2601 Sedgwick Ave Bronx NY